[ Up ] [ noninteracting discrete species ] [ self-association ] [ Nonideal Sedimentation ] [ c(M) ] [ c(M) with other prior ] [ c(s) ] [ c(s) with other prior ] [ c(s,M) and c(s,*) ] [ ls-g*(s) ] [ van Holde-Weischet ] [ ls-g*(s) with 1 discrete LE component ] [ extrapolate ls-g*(s) ] [ Analytical Zone Centrifugation ] [ DLS ] [ electrophoresis and other geometries ] [ Special ]

ls-g*(s) distribution with 1 discrete Lamm equation component

This is an extension of the ls-g*(s) model.  Please see this page first. 

The additional feature of this model consists in the possibility to consider a single diffusing species in addition to the distribution.  The distribution is still an apparent sedimentation coefficient distribution, without consideration of diffusion.  However the extra species allows for modeling, for example, the sedimentation profiles of relative small molecules that exhibit significant diffusion on the time-scale of sedimentation.

This is an example of a small protein (Mw = 20kDa) co-sedimenting with a broad distribution of large protein complexes with Mw > 1MDa.  It is very useful to take the sedimentation and diffusion of the small species precisely into account, while the approximation of no diffusion remains valid for the large species.

The concentration of the discrete will be saved as the first entry in the distribution.  (The distribution plot in the above graphics is rescaled to omit this species.)

The parameter box is the same as the ls-g*(s), but with the s-value and molar mass of the extra species to be entered in the upper right corner.  These parameters can also be fitted for.

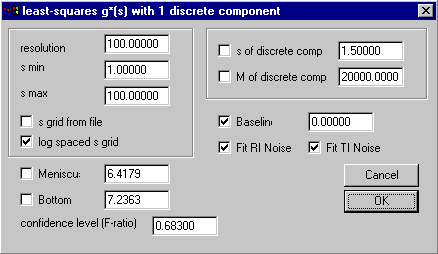
This is very analogous to the c(s) model with extra discrete species.