Concentration Dependent Sedimentation
Model | Nonideal Sedimentation
This model is for a single non-ideal sedimenting component. (A non-ideal component in the presence of other ideally sedimenting components is possible from version 8.5, see below). Hydrodynamic and thermodynamic nonideality is taken into account in a linear approximation as
s = s0/(1+ks x c) and D = D0 (1+ kd x c) for the repulsive case, and
s = s0/(1-ks x c) and D = D0 (1 - kd x c) for attractive nonideality,
where s0 and D0 are the sedimentation and diffusion coefficients at infinite dilution (uncorrected for density and viscosity), and ks and kd are non-ideality coefficients. Details of the numerical procedures of the finite element routine are described here. Numerical accuracy was tested with a limiting case described by Ref 1.
From both non-ideality coefficients, the second virial coefficient can be calculated as kD » 2 A2M - ks (Ref 2). The application of the model with SEDFIT and SEDPHAT is described in Ref 2, also including a general strategy how to use this model best.
The parameter box has many of the common parameters described in the independent species model. All model-specific parameters are nonlinear, and optimized only in a fit command.

The first model-specific field is for the total loading concentration (in signal units). Below is the field for the sedimentation coefficient at infinite dilution (uncorrected for density and viscosity), and its nonideality coefficient. The diffusion coefficient at infinite dilution follows and the respective nonideality coefficient.
The input of both coefficients ks and kd is in the form of the base 10 logarithm of this parameter (i.e. -2 for 0.01). The units of these coefficients are inverse signal units (e.g. 1/OD280 or 1/fringe), and for further interpretation should be converted by using appropriate extinction coefficients (taking into account the pathlength 1.2 cm) or refractive index increments.
The concentration dependence can be either due to repulsive or attractive interactions. In the repulsive case (default, mark in the checkbox 'repulsive'), ks and kd are positive, whereas the attractive case (checkbox 'repulsive' not marked) is implemented where both ks and kd are negative.
This function will be further extended shortly and implemented in for global modeling in SEDPHAT.
Please note: Starting from version 8.5, there is a new option of using arbitrary polynomial expressions or s(c) and D(c).
This provides more freedom for describing highly concentration dependent sedimentation, where the usual corrections with ks and kd do not hold anymore. Examples are the sedimentation of CsCl at concentrations that establish significant density gradients. The units for the concentration are signal units (i.e., interference fringes, or absorbance values), except for the CsCl data, which are hardwired and taken from Allen Minton's paper in Biophys. Chem. 42 (1992) 13-21. For the polynomials, Sedfit reads two files "c:\sedfit\snonid.dat" and "c:\sedfit\dnonid.dat" , which are simply a 1 column ASCII text file with the polynomial coefficients. The number of coefficients is not predetermined, and will simply be taken from the file.
Also, Starting from version 8.5, a non-ideal species can be modeled in the presence of up to three ideally sedimenting species. To do that, switch to the ideal independent species model, and make sure the fitting parameters are set to s and D. Then, the parameter box will look like this:
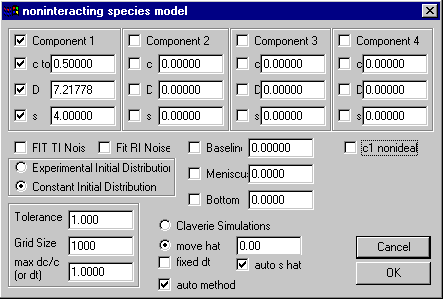
Notice the "c1 nonideal" switch on the right below the parameter sections. If this is switched on, component 1 will sediment nonideally, and the user will be prompted to enter the non-ideal parameter ks and kd, and specify if they are fixed or should be fitted for.
New in version 8.7: The concentration-dependence of the sedimentation coefficient from hydrodynamic non-ideality has been combined with the concentration dependence of s in a rapid monomer-dimer self-associating system.
References
1) Dishon, M., G. H. Weiss, and D. A. Yphantis. 1967. Numerical solutions of the Lamm equation. III. Velocity centrifugation. Biopolymers. 5:697-713.
2) Solovyova, A., P. Schuck, L. Costenaro, C. Ebel (2001) Non-ideality by sedimentation velocity of halophilic malate dehydrogenase in complex solvents. Biophys. J. 81:1868-1880